Multiplication-Napier bones method

Multiplying a two-digit number with another two-digit number would require a 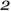 by 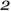 grid, for example .

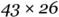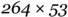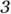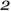Multiplying  would require a  by grid.

Follow the steps below to see how Napier’s method is used to calculate .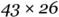

• The first step is to draw a  by  grid.
• The second step is to draw a diagonal in each box. The diagonal line separates the tens and the units. Always write the tens above the diagonal line in each box.
• Start by multiplying  and  to fill the left box on the top row.
• 
• Write  and  to show that there are no tens.

• Now multiply  and  to fill the right box on the top row.
• 

• Next, multiply  and  to fill the left box on the bottom row.
• 
• Remember to put the  above the diagonal.

• Complete the grid by multiplying  and  to fill the right box on the bottom row.
• 

After completing the grid, add the columns along the diagonals, starting at the bottom-right. We sometimes need to carry over from one diagonal to the next. To get the answer, read the totals down the left and to the right.

REFERENCE FROM https://www.bbc.co.uk/education/guides/zvvg87h/revision/2

Q